ジグザグ補題

数学、特にホモロジー代数学におけるジグザグ補題（ジグザグほだい、英: zig-zag lemma）は、鎖複体のホモロジー群から成るある種の長完全列の存在を述べるものである。この結果は任意のアーベル圏で通用する。

任意のアーベル圏（アーベル群の圏や与えられた体上のベクトル空間の圏など）において、${\displaystyle ({\mathcal {A}},\partial _{\bullet }),({\mathcal {B}},\partial _{\bullet }'),({\mathcal {C}},\partial _{\bullet }'')}$ が以下の短完全列を満たす鎖複体だとする：

${\displaystyle 0\longrightarrow {\mathcal {A}}{\stackrel {\alpha }{\longrightarrow }}{\mathcal {B}}{\stackrel {\beta }{\longrightarrow }}{\mathcal {C}}\longrightarrow 0}$

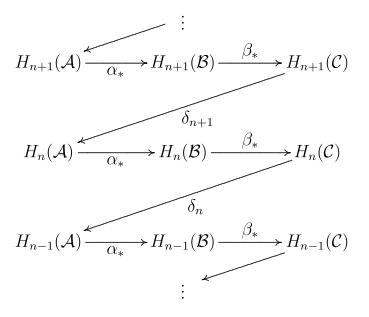
この系列は以下の可換図式の略記であるとする：

ここで各行は全て完全で、各列は全て鎖複体である。

ジグザグ補題は、境界写像（族）

${\displaystyle \delta _{n}:H_{n}({\mathcal {C}})\longrightarrow H_{n-1}({\mathcal {A}}),}$

が存在して、次の系列を完全にすることができることを主張する：

${\displaystyle \alpha _{*}^{}}$ と ${\displaystyle \beta _{*}^{}}$ は、通常のやり方で誘導されたホモロジー群の間の写像である。境界写像 ${\displaystyle \delta _{n}^{}}$ は以下の節で説明する。この補題の名称は、系列における写像が「ジグザグ」に走ることから来ている。不運な用語法のバッティングにより、ホモロジー代数には『蛇の補題』の名を持つ別の結果があるにもかかわらず、この命題（ジグザグ補題）はその名（蛇の補題）でも一般に知られている。蛇の補題を使うと、ジグザグ補題のここに記すものとは別の証明が得られる。

写像 ${\displaystyle \delta _{n}^{}}$ は標準的な図式追跡の議論を使って定義できる。${\displaystyle c\in C_{n}}$ を、 ${\displaystyle H_{n}({\mathcal {C}})}$ に属すある同値類の代表元とする。よって ${\displaystyle \partial _{n}''(c)=0}$ 。行方向の完全性より ${\displaystyle \beta _{n}^{}}$ は全射なので、${\displaystyle \beta _{n}^{}(b)=c}$ となる ${\displaystyle b\in B_{n}}$ が存在しなければならない。図式の可換性より、

${\displaystyle \beta _{n-1}\partial _{n}'(b)=\partial _{n}''\beta _{n}(b)=\partial _{n}''(c)=0}$

再び行方向の完全性より、

${\displaystyle \partial _{n}'(b)\in \ker \beta _{n-1}=\mathrm {im} \alpha _{n-1}}$

${\displaystyle \alpha _{n-1}^{}}$ は単射だから、${\displaystyle \alpha _{n-1}(a)=\partial _{n}'(b)}$ を満たす ${\displaystyle a\in A_{n-1}}$ が一意的に存在する。これは輪体である。なぜなら ${\displaystyle \alpha _{n-2}^{}}$ は単射で、かつ ${\displaystyle \partial ^{2}=0}$ より

${\displaystyle \alpha _{n-2}\partial _{n-1}(a)=\partial _{n-1}'\alpha _{n-1}(a)=\partial _{n-1}'\partial _{n}'(b)=0}$

が従うからである（つまり ${\displaystyle \partial _{n-1}(a)\in \ker \alpha _{n-2}=\{0\}}$ ）。${\displaystyle a}$ は輪体なので、${\displaystyle H_{n-1}({\mathcal {A}})}$ に属すある同値類の代表元になる。ここで、

${\displaystyle \delta _{}^{}[c]=[a]}$

と定義する。このように定義された境界写像は well-defined であることが示せる（つまり写像が c と b の選択に依らずに定まる。証明は上記の図式追跡の議論と同様である）。また同様の議論で、長系列が各ホモロジー群のところで完全であることも示せる。

• マイヤー・ヴィートリス完全系列

• Hatcher, Allen (2002). Algebraic Topology. Cambridge University Press. ISBN 0-521-79540-0. http://www.math.cornell.edu/~hatcher/AT/ATpage.html 
• Lang, Serge (2002), Algebra, Graduate Texts in Mathematics, 211 (Revised third ed.), New York: Springer-Verlag, ISBN 978-0-387-95385-4, MR1878556, https://books.google.co.jp/books?id=Fge-BwqhqIYC 
• Munkres, James R. (1993). Elements of Algebraic Topology. New York: Westview Press. ISBN 0-201-62728-0